g Anisotropy
When dealing with magnetic moments in a crystalline or molecular environment one has to be aware that the CW and pulse EPR spectra may either depend on the orientation of the sample in the magnetic field \( \mathbf{B}_0 \) (e.g. single crystals) or are superpositions of many different single crystal spectra with random orientation (e.g. powder samples). In solution rotational and translational motion of the molecules often averages out the anisotropic interactions. Due to short relaxation times pulse EPR measurements have to be performed at low temperatures where molecular motion is frozen. These `frozen solutions' EPR spectra are qualitatively identical to powder spectra. Thus the concept of anisotropy is of importance and we will discuss the anisotropy of the EZI in more detail.
In the first example the only magnetic field experienced by the electron spin was the external magnetic field \( \mathbf{B}_0 \) and the spin vector \( \mathbf{S} \) was oriented either parallel or antiparallel to \( \mathbf{B}_0 \). As shown above additional inner fields, transmitted to the electron spin by the SOC, are present in molecular systems which may be larger than the external field. These fields are related to the symmetry of the molecule, e.g. to the CF of a transition metal ion. The effective field \( \mathbf{B}_\text{eff} \) experienced by the electron spin in a molecular or crystalline environment, is a superposition of the external and internal fields. For an anisotropic system Eq. (1) is written as:
$$ \mathcal{H}={g}_e\frac{\beta_e}{h}\mathbf{B}_{\text{eff}}\mathbf{S} = \frac{\beta_e}{h} \mathbf{B}_0 \mathbf{g} \mathbf{S} \qquad (4) $$
In the second part of Eq. (4) the orientation dependent variation of the Zeeman splitting is expressed by a (3x3) g interaction matrix. The information about the symmetry of the inner fields is thus transferred to a g matrix and can be obtained experimentally by determining the principal values of this matrix. In general the g matrix will be orthorhombic and is expressed in its principal axes system (PAS) by
$$ \mathbf{g} = \left[ \begin{array}{ccc} {g}_x & {} & {} \\ {} & {g}_y & {} \\ {} & {} & {g}_z \end{array} \right] \qquad (5)$$
where (x,y,z) is the PAS and gx, gy and gz are the principal values of g. For B0 along one of the principal axes, the electron spin vector is quantized along B0 and the resonant field is obtained by inserting the corresponding principal value into Eq. (3). For an arbitrary orientation of B0 the expression for the resonant field is obtained by insertion of the following expression into the resonance condition:
$$ \text{g}^2 = {g}^2_x l ^2_x + {g}^2_y l ^2_y + {g}^2_z l ^2_z \qquad (6)$$
where lx, ly and lz are the direction cosines between B0 and the three principal axes (x,y,z).
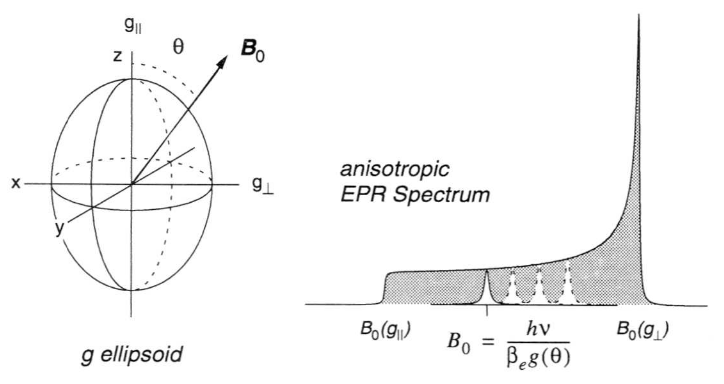
To illustrate the effect of g anisotropy on the EPR spectrum we consider the special case where the CF has axial symmetry (e.g. a transition metal ion in octahedral coordination with a tetragonal distortion along the z axis). In this case the EZI is described by two parameters g⊥ and g|| given by
$$ {g}_\bot = {g}_x = {g}_y \qquad (7a)$$ $$ {g}_\parallel = {g}_z \qquad (7b)$$
Eq. (6) can now be rewritten with a single angle θ between the magnetic field vector B0 and the z axis.
$$ \text{g} (\theta)^2 = {g}_\bot^2 \sin ^2 \theta + {g}^2_\parallel \cos ^2\theta \qquad (8)$$
In Fig. 3 an axial g matrix with g|| > g⊥, represented by a rotational ellipsoid, and the line shape of the corresponding EPR spectrum are drawn, assuming a large number of paramagnetic systems with random orientation of their g ellipsoids with respect to the static magnetic field B0. This situation is typical for a powder sample. For a given magnetic field strength B0, all spins fulfilling the resonance condition g(θ) = hν/βeB0, i.e. all spins for which B0 makes an angle θ with the z-axis of the g ellipsoid, contribute to the spectrum and are considered to form a spin packet. These spin packets are schematically exemplified in the powder line shape in Fig. 3. The extreme positions of the powder spectrum are obtained by inserting g|| and g⊥ into the resonance condition. Note that for g|| > g⊥ one obtains B0(g||) < B0(g⊥) due to the inverse proportionality of g and B0. The asymmetric line shape is mainly due to the fact that the number of spin packets contributing to the spectrum is much larger in the xy-plane than along the z- axis.
The case discussed above is typical for the Jahn-Teller distorted Cu2+ complex in octahedral symmetry. The degeneracy of the ground state in the octahedral coordination field is removed either by an elongation or a compression along the z-axis. The axial symmetry of the g matrix thus reflects the symmetry of the ligand field.